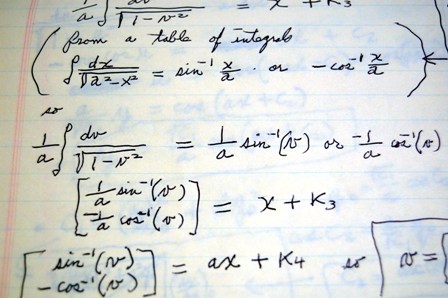In a moment, this will turn into a post about math, but let’s start with foreign languages. There’s a moment when we, as tutors, are classically called in to help a student struggling with a foreign language. Often some time in year two, occasionally late in year one, out of nowhere, a student who’s been getting solid grades starts struggling.
For an experienced tutor the diagnosis is usually pretty simple, if the solution is not. It’s a matter of critical mass. Many students spend the first year or so successfully staying afloat, quiz to quiz, mini-dialog to mini-dialog, handling each word list and verb conjugation as it arrives. The trouble is, at a certain point, the poor kid can’t keep track of it all. The balls all drop. The budding linguist focused too much on the mastering each chapter on its own terms and didn’t invest the extra effort in finding continuity in the material. Sometimes the crisis arises when the student simply reaches saturation, with too many discrete concepts to hold onto. Sometimes it comes when expectations shift and suddenly the homework and tests demand that the student be able to take all of those seemingly independent word lists, conjugations, and gendered endings and make them work together, all at once. The wheels come off; grades plummet; everybody panics.
There’s a lesson in this not only for students (and parents) in crisis over Spanish, French, or Japanese. We often see a similar pattern develop in students struggling with math, Algebra, in particular. Where in languages, problems arise for the student who over-relies on flashcards, in math, it’s the “plug-and-chugger” who loses the thread. Students who were once able to get by, over time, start to founder often in the second semester of Algebra 1 or early in Algebra 2. In important respects, the problem really is a language issue. It’s an old commonplace that math is itself a foreign language, but too often teachers and parents don’t think about what that really implies about how it’s best taught.
We’ve found that math teachers and curricula at schools all around the city don’t spend enough time on the continuity of math operations and the fundamental grammar of the concepts and techniques they teach. For example, FOIL is an excellent mnemonic for how to distribute when multiplying two summed quantities, but often the mnemonic is left to do too much of the work. Students aren’t reminded how the basic principles of arithmetic (the old Associative, Commutative, and Distributive Properties, for example) still describe what the operation does, or how FOIL helps as a work-around for the Order of Operations (itself known more for its acronym, PEMDAS, than its underlying logic). Without a grounding in the fundamental principles, students can’t know how to generalize the technique and adapt it to new circumstances. Instead, FOIL is taught as its own thing; exponent rules are taught as their own thing; geometric growth is taught as its own thing. Students jump from chapter test to chapter test, quiz to quiz, mnemonic device to mnemonic device. And soon, we’re back to virtually the same crisis we were talking about before.
When we, as tutors, come into this scene with a foreign language student, our task is clear: we have to rebuild the foundations, from the basic grammar to the vocabulary, integrating as we go. We all have our strategies. We explain, perhaps, that we’re going conduct sessions solely in the language of study because it reveals the ongoing relevance of old grammar and old vocabulary. We encourage students to write compositions or phrase answers to this week’s homework assignments using last week’s and last month’s grammar and vocabulary as much as possible. In time, we help the student learn how to embed new material as it comes along in the old lessons he or she learned. Everything mutually reinforces.
Often, in math tutoring, we take the same approach. We back off the current concept and try to reestablish the context. We urge students to talk through the skill, trying to locate this lesson’s place in the grammar of math, other operations, other concepts. It’s always there; the student just has to find it. Many are quick to label – good math students versus bad ones, kids with an ear for languages and those who can’t get the hang – but, all too often, the difference is one of emphasis and perspective. Understanding may not be too far off and the answers might lie in the things learned long ago.
